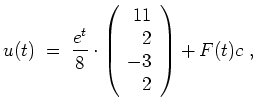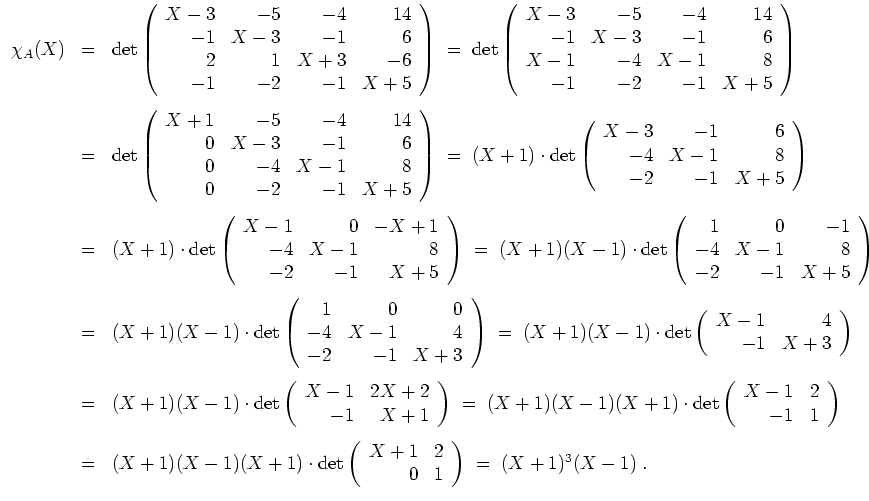
Es wird
Beim Eigenwert
![]() erhalten wir
erhalten wir
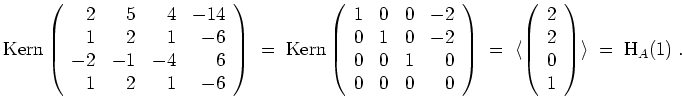
Beim Eigenwert
![]() setzen wir zunächst
setzen wir zunächst


Ferner wird
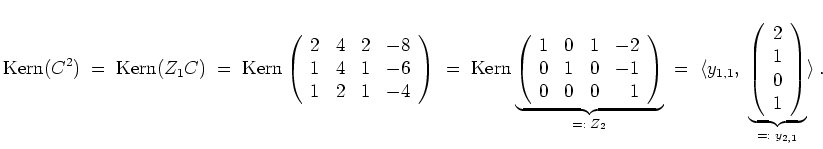
Schließlich wird
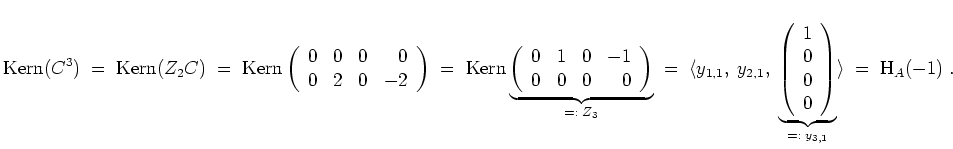
Wählen wir
![]() , so sind schon aus Dimensionsgründen in den Stufen
, so sind schon aus Dimensionsgründen in den Stufen
![]() und
und
![]() keine weiteren Vektoren auszuwählen. Der Eigenwert
keine weiteren Vektoren auszuwählen. Der Eigenwert
![]() liefert also die Kette
liefert also die Kette
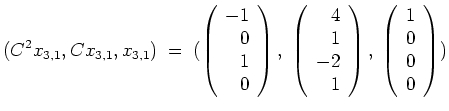
Insgesamt erhalten wir mit
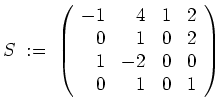
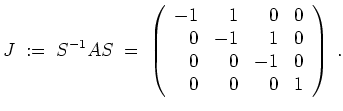
Somit erhalten wir
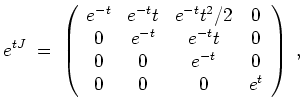
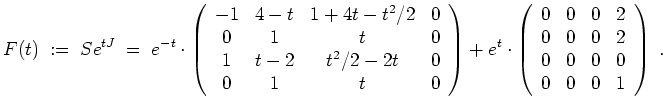
Bestimmen wir nun eine partikuläre Lösung der inhomogenen Gleichung. Hierzu berechnen wir zunächst